Chemical Physics

Theoretical Background

Wetting is the process of making contact between a solid and a liquid. Controlling the wettability of solid materials is a classical and key issue in surface engineering. A few examples of wetting-dependent processes important in daily life, biology and industry include adhesion, printing, cleaning, painting and lubrication.

Most processes that involve liquids deal with situations where the free surface of the liquid meets a solid boundary, thus forming the so-called three-phase contact line; this is the line where solid,liquid and gas meet. The contact line can move along the solid surface, leading to "wetting" or "dewetting". The interaction between a liquid and a solid involves three interfaces: the solid-liquid interface, the liquid-vapor interface and the solid-vapor interface. Each of these interfaces has an associated surface tension, γ, which represents the energy required to create a unit area of that particular interface. A different approach is to regard γ as a force acting on the drop. This approach is shown in Figure 1, where γ appears as an arrow. At equilibrium, force equilibrium along the X axis provides a relation between the angle, θ, and the surface tensions of the three interfaces. This is called Young's equation:

$$\gamma_{SG} = \gamma_{SL} + \gamma_{LG}cos(\theta)$$

where γ_SG, γ_SL and γ_LG are the surface tensions of solid/gas, solid/liquid and liquid/gas interfaces, respectively. θ is the angle between a liquid drop and a solid surface, called the contact angle.

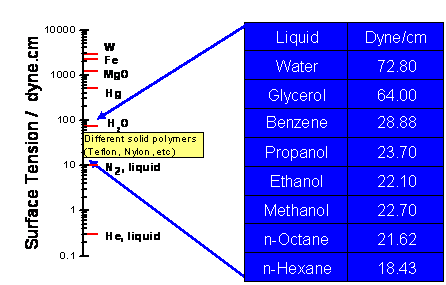
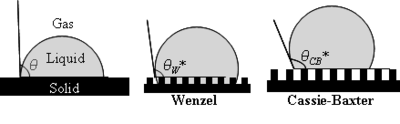
The magnitude of Young's contact angle is the result of energy minimization. If the liquid-gas surface tension is smaller than the solid-gas surface tension (γ_LG < γ_SG), the liquid-solid interface will increase to minimize energy. As the drop wets the surface, the contact angle approaches zero, leading to complete wetting. Other ratios of γ_LG and γ_SG will lead to the formation of drops of different shapes as shown in Figure 1. A hydrophilic surface is defined as a surface where 0°<θ<90°, and a hydrophobic surface is a surface where θ≥90°. The surface tensions of different substances in contact with the gas phase vary over a wide range is shown in Figure 2.

Different shapes of drops on a solid. The surface tensions, γ values, associated with each interaction interface are shown by red arrows. The contact angle θ between the liquid drop and the solid surface is also shown.

Comparison of surface tension of different materials in contact with their vapor.

Interactions between Surfaces and Liquids

In order to understand why different surfaces and liquids form different contact angles, we must consider the interaction between the liquid and the surface and between the liquid molecules themselves. The adhesive force between the surface and the liquid causes the drop to spread and wet the surface, and the cohesive force within the liquid drop causes it to ball up and avoid contact with the surface. For example, let's consider the contact angles between water and three types of surfaces - polymer, metal and oxide.

Some polymers, such as PVC and Teflon, form mainly Van der Waals interactions with a water drop placed on them. These interactions are weak relative to the hydrogen bonds within the drop, so that the water molecules interact mainly among themselves and not with the surface. The result is a bead shaped drop that almost does not wet the surface. These are hydrophobic surfaces.
Oxide surfaces can form hydrogen bonds with the water molecules in the drop. These bonds apply strong forces on the interface, and so the water tend to wet the surface rather than ball into a bead. These are hydrophilic surfaces.
Metal surfaces do not form strong hydrogen bonds with water; however their polarity is greater than that of PVC or Teflon, so their affinity to water is stronger. The result is that their contact angle is usually between that of the polymer surfaces and oxide surfaces.

The same considerations can be used to predict the contact angles of an organic liquids such as oil on different surfaces.

Controlling the Contact Angle

The surface tension and contact angle can be controlled through different methods.

Temperature: Generally, surface tension decreases as the temperature increases. The dependence of surface tension on temperature is usually approximately linear reaching zero at the critical temperature (Figure 3).

Surface tensions of water and benzene at different temperatures.
Surface roughness: Wenzel's model states that if the surface is rough and water penetrates into the roughness, whatever trend the flat surface showed will be enhanced. For example, a hydrophobic surface will become even more hydrophobic and a hydrophilic surface will become more hydrophilic as the surface roughness increases. According to the Cassie and Baxter model, the water drop does not penetrate into the surface grooves and instead lies on top of them, so that air bubbles are trapped between the surface and the water. The trapped air increases the contact angle and the surface becomes superhydrophobic. Both models are shown in Figure 4.

The effect of surface roughness on the contact angle as shown from left to right for a smooth surface, a rough surface that obeys Wenzel’s model, and a rough surface that obeys the Cassie-Baxter model (with air, in white, shown trapped between the liquid and the solid).

Electro-wetting: When a potential difference is applied between the liquid and the solid surface, the electric force at the edges of the drop will pull it down onto the surface, lowering the contact angle.
Chemical modifications: Chemical modification of the surface can lead to a change in its surface tension. For example, adding polar, hydrophilic groups to the surface will lead to a lower contact angle. The next section gives two concrete examples of such chemical modifications.

Surface Modifications

A metal surface is usually hydrophilic, especially if it is oxidized. A pure metal surface exhibits a strong interaction with the oxygen of water molecules (Figure 5a), whereas an oxidized surface forms hydrogen bonds with water (Figure 5b).

a	b

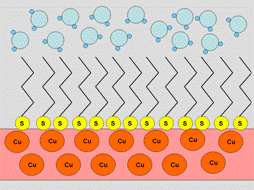
Schematic view of (a) metal/water interface and (b) oxide/water interface

The most common way to make a surface hydrophobic is to modify it with sulfur-containing, HS-R, where R is an alkyl with more than six repeating units ((-CH₂)n) in the chain. The interaction of these substances with a copper surface can be described as follows:

$$CuO + 2H-S-R\Rightarrow Cu-(S-R)_2 + H_2O$$

$$Cu(OH)_2 + 2H-S-R \Rightarrow Cu -(S-R)_2 + 2H_2O$$

$$Cu + 2H-S-R \Rightarrow Cu-(S-R)_2 + H_2$$

$$Cu + (S-R)_2 \Rightarrow Cu-(S-R)_2$$

As a result of these reactions the metal surface is blocked from interacting with solvent molecules by the alkyl chains, replacing the strong metal-water interactions with weak interactions between water with saturated alkyl chains. If, instead of sulfur-containing alkyls, one uses substances like HS-R-X or (S-R-X)₂, where R is a long alkyl chain and X can form hydrogen bonds (e.g., NH, NH₂, SO₂), then the modified surface will be hydrophilic.

a	b	c

(a) disulfide and (b) thiol groups. (c) shows the metal/alkyl/water interface

Effect of Surfactants on the Surface Tension of Water

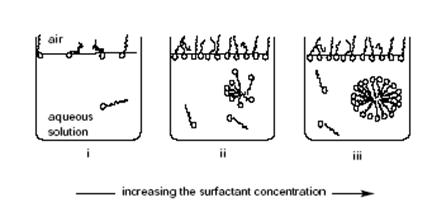
Surfactant molecules accumulate preferentially at the liquid-vapor interface with the polar groups oriented toward the aqueous phase (Figure 7i). This positive adsorption of surfactant molecules lowers the liquid-vapor interfacial energy, and consequently the surface tension decreases. The surface tension continues to fall as the surfactant concentration increases until the critical micelle concentration is attained. At this stage the adsorbed molecules are closely packed, forming an oriented monomolecular layer (monolayer) (Figure 7ii). Above this concentration, additional surfactant molecules aggregate to form micelles in the bulk. This cooperative association process continues in the bulk with further increase of surfactant concentration, while at the surface of the solution the molecules remain in a highly condensed state. The concentration of free surfactant is essentially constant as the micelle concentration increases (Figure 7iii); free surfactant and micelles exist in dynamic equilibrium. The surface tension remains approximately constant for the systems studied since it depends only on the liquid-vapor interfacial structure. The same type of behavior is found for the magnitude of the contact angle; that is, as the surfactant concentration increases, the contact angle decreases until a constant value is attained. For higher concentrations the contact angle remains approximately constant.

Schematic arrangement of surfactant molecules in aqueous solution: (i) at low concentrations- the surfactant molecules adsorb preferentially at the air-water interface; (ii) at intermediate concentration a monolayer is formed at the air-solution interface; (iii) at high concentration the state of surface is unchanged, and surfactant molecules aggregate as micelles.

Zisman Plot

The surface tension at a solid/gas interface can be determined from a Zisman plot, which measures variation in contact angle as a function of the known surface tension, γ_LG, for a series of liquids. The experimentally obtained dependence of cos(θ) on γ_LG is often linear. The value for which cos(θ) extrapolates to 1 is termed the surface tension, γ_SG , of the solid/gas interface. A typical Zisman plot is shown in Figure 8.

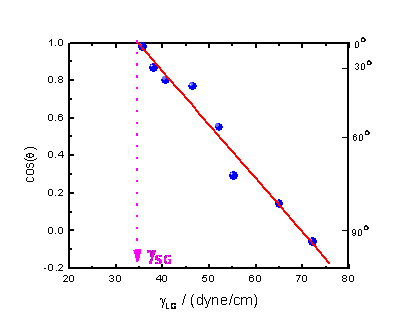
The idea of this approach is as follows: a liquid with a surface tension less than or equal to the surface tension of a particular material will wet that surface (i.e., the contact angle will be 0°). This widely used approach can be applied only under proper conditions, however.
The Young equation at θ = 0 gives:

$$\gamma_{SG} = \gamma_{SL} + \gamma_{LG}$$

In contrast, by the Zisman approach, γ_SG = γ_LG. In other words, the liquid and solid studied must have an interface with γ_SL≈0. This approximation is accurate in the case of organic polymer solids and liquid saturated hydrocarbons or aqueous solutions of saturated alcohols at sufficiently high concentrations (>20%). This is the reason why the surface tension obtained from a Zisman plot is often referred to in the literature as the critical surface tension of wetting but not the surface tension of a solid/gas interface.