Vacuum Techniques
In this experiment you will learn about vacuum technology and how to use it for measurements.
Experimental procedure
Determine the atmospheric pressure by measuring the force needed to open the vacuum system
- Build the illustrated system:

Figure 1a: Schematic illustration of the system to be built to measure the force needed to open the vacuum system. 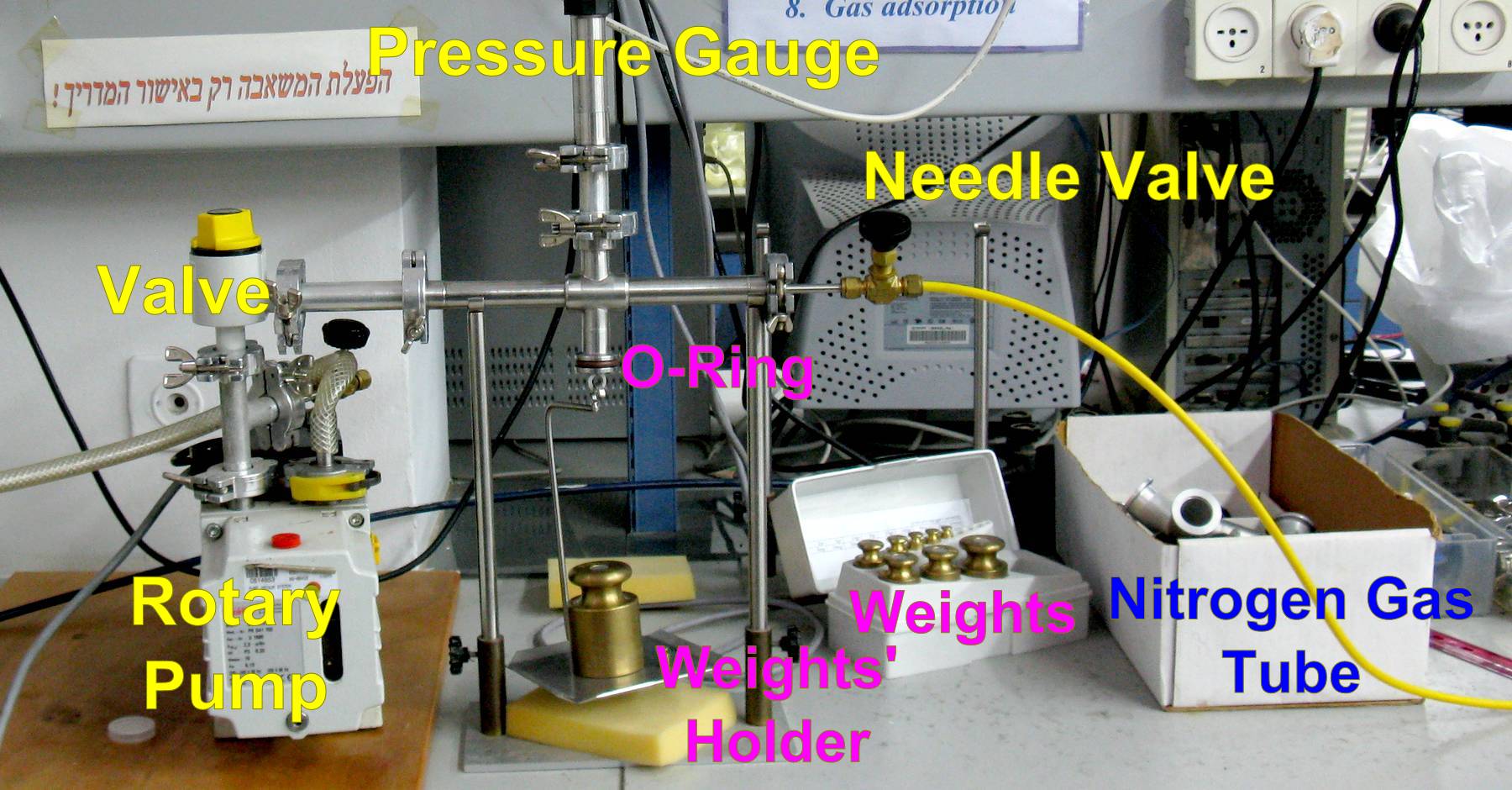
Figure 1b: Photograph of the same system illustrated in Figure 1a. - Hold the weight holder, and press it against the O-ring.
- Pump the air from the system, making sure that none of the connectors leak.
- Once the system is under reduced pressure, close the valve connecting the system to the pump.
- Calculate the expected pressure in which the weight will disconnect before performing the experiment.
Weight [gr] Weight Error [mg] Pressure 1000 290 --- 500 100 --- 100 30 --- 50 30 --- 20 20 --- 10 20 --- 5 10 --- - Let the nitrogen flow slowly through the needle valve that is directly connected to the vacuum system until the weight falls. Monitor the pressure of the system and record the pressure immediately before the weight falls. Based on this first measurement and your calculation, what are likely sources of error in this experiment? Estimate their values and write them down.
- Repeat this experiment for several weights.
- Fill in the table and plot (in the correct units) pressure versus total weight. Find the atmospheric pressure and find the O-ring sealing area.
| Total Weight | Pressure |
|---|---|
| --- | --- |
Measurement of pumping speed of the rotary pump when volume is fixed
- Build the system illustrated in Figure 2. Notice that you should build your system as large as possible (why should you do this?). Before building the system, measure the dimensions of all components so that you can estimate your system volume via direct measurements.

Figure 2a: Schematic illustration of the system to be built to measure the pumping speed of the rotary pump when the volume is fixed. 
Figure 2b: Photograph of the same system illustrated in Figure 2a. - Make sure that both the Pirani and the Piezo pressure gauges are connected.
- In order to measure the pumping speed of the pump, you should first measure the volume of your system using a known volume. In this case, of the cylinder. Assuming the gases are ideal and moles are conserved, by the use of Boyle's law, the unknown pressure can be calculated by the expression:
The measurement process:
$P_{total} V_{total}=P_{known} V_{known} +P_{unknown} V_{unknown}$
$V_{total}=V_{known} + V_{unknown}$
$(P_{known}-P_{total})V_{known}=(P_{total}-P_{unknown})V_{unknown}$
- Construct the illustrated system and close all the valves. The system will be at atmospheric pressure.
- In order to measure the unknown volume of your system, you will need to measure both the pressure of your system (the unknown volume) and of the cylinder (the known volume), at every time that you open or close one of the valves.
- You can start either from atmospheric pressure or from vacuum. To start from vacuum, first pump down the entire system. Write down the pressure you obtained and its error as $P_{known}=P_{eq}^{(1)}$.
- o With the two parts of the system still separated : If you started from atmospheric pressure open the pump needle valve and pump down the unknown volume. If you started from vacuum, open the nitrogen needle valve and allow it to flow to the unknown volume. Close the needle valve that you opened. Write down the new pressure, $P_{unknown}^{(1)}$, and its error (note that the pressure in the cylinder is still $P_{eq}^{(1)}$).
- Open the valve separating the two parts of the system (the known and the unknown volumes) and wait until it stabilizes. Write down the pressure, $P_{total}=P_{eq}^{(2)}$, and its error.
- Close the valve separating the two parts and ether open the pump needle valve and pump down the unknown volume or open the nitrogen needle valve and allow it to flow to the unknown volume. As before take a measurement of the pressure in your system, $P_{unknown}^{(2)}$, and its error (note that the pressure in the cylinder is still $P_{eq}^{(2)}$).
- Repeat the process.
- You should fill the table (make sure the correct units are used):
$$P_{eq}$$ $$P_{unknown}$$ --- --- - Plot $P_{eq}$ versus $P_{unknown}$, and determine the volume of the system and its error.
- Compare this result to the estimated volume of your system based on measurements of components made at the beginning of the experiment.
- After you have measured the system volume, pump down the entire system and then close both the needle valve of the pump and the valve separating the two parts of the system.
- Allow nitrogen to flow only to your vacuum system and fill it until reaching atmospheric pressure. Do not pass the 1 atmosphere limit!.
- Begin taking a video of both the Pirani and the Piezo pressure gauges. Open the pump's needle valve and pump nitrogen out of the system. Note: The pressure gauges are connected and each gives a readout. Considering the gauges working pressure ranges and the dependence of the pumping speed on the pressure, is one of the gauges more correct (consider the measurement ranges provided by the manufacturer)? Which one? Monitor both readouts to defend your answer.
- Plot a graph that will allow you to calculate the pumping speed, S. Note that you should have at least one measurement per second. Compare your results to the manufacturer's value.
- Repeat steps 5-8, but this time, first open the valve separating the two parts of the system, and allow nitrogen to flow to the entire vacuum system until reaching atmospheric pressure. Compare the results.
Measurement of pumping speed of the rotary pump when pressure is fixed
- Disassmeble the system from the previous part and build a new system similiar to that illustrated at Figure 2a (without connecting your system to the known volume or to the Nitrogen gas source) and connect it to the mass flow meter (MFM). Make sure that the needle valve of the MFM is closed.
- In this part (and the following) use atmospheric pressure as your "source". Given that the pressure gauges are calibrated to measure nitrogen gas, will this affect the accuracy of readouts? Think carefully.
- Gently open the needle valve of the MFM and allow air to flow in. Wait for the reading to stabilize.
- Measure the pressure in your system and the rate of gas flow into your system. Be careful to take readings only from an instrument that is within range!
- Determine the pumping speed, S, and compare it to the value you obtained in the previous section. Compare your result to the manufacturer's value.
Measurement of conductivity of different tubes and calculation of the viscosity of air
At your disposal there are tubes of different lengths and inner diameters.
- Build the system illustrated in Figure 3 with one of the tubes:

Figure 3: Schematic of system to be used to measure conductance of a tube. - Allow air to flow into the system via the needle valve connected to the MFM.
- Note the MFM reading and both of the pressure gauges readings. Make sure that you are in the linear range of all the gauges.
- Repeat this experiment with each tube. Fill the table below with your data (use the correct units).
Tube Diameter Tube Length SMass Flow Meter PGauge 1 PGauge 2 ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- ----- - Is it possible to determine the conductivity for each tube? What is the relavant equation?
- If not, why not?
- See equation 14, does the conductivity remain constant?
- Compare your results for conductivity of the tubes using equation (14). Take the air viscosity value from literature.
- What type of flow does eq.14 describe? Did you measure this type of flow?
- For each tube conductivity, you should be able to calculate the viscosity of the air. Compare the calculated air viscosity with the air viscosity value from literature.
- Using results obtained from experiments with different tubes, demonstrate that gas flow rate depends on the tube's diameter raised to the fourth power as stated in Poiseuille’s law (equation (13)). For this plot you will need to have at least five points (i.e., 5 measurements of tubes with the same length but different diameters).