Answer to the Question 10/00
CHARGES ON A CIRCLEThe question was:
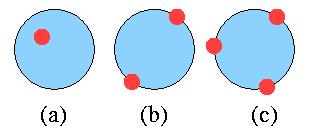
Several identical point charges (shown in red) are placed on a circular disk (shown in blue) so as to minimize the electrostatic energy of the system. Obviously, single charge can be placed anywhere as depicted in Fig. (a), while a pair of charges will occupy opposite sides of the diameter of the circle, as in Fig. (b). Similarly, three charges will form an equilateral triangle, as in Fig. (c). What can you say about the geometric arrangement of 4, 5, 6, ... charges?
(1/2003) We did not receive an answer to the question. However, Karthik Tadinada brought to our attention a paper by K.J. Nurmela (a postscript file of this work can be found here), where a detailed study of cases containing up to 80 charges has been performed. The paper also contains numerous references to other works on the subject.
The answer as we know it so far:
For number of charges n<12, the minimum energy configuration consists of charges spread out on the boundary. For n=12,13,14,15 and 16, we get one charge in the center and the remainder on the boundary. For n=17 and 18 already two charges are inside the circle. As the number of charges continue to increase, the positions of the charges resemble more and more Wigner crystal (triangular lattice) of charges. As the number of charges approaches infinity, the mean density of the charge distribution approaches the known density of continuous charge distribution on a circle (density ~ 1/sqrt(1-r2), where r is the distance from the center of the disk).
 Back to "front page"
Back to "front page"